Let's Make a Deal!

While reading the very sporadic and entertaining, The Curious Incident of the Dog in the Night-Time, by Mark Haddon, a book written from the perspective of a boy with Asperger's Syndrome who is trying to solve a crime, I came across the Monty Hall problem. This problem was illustrated in the old game show Let's Make a Deal. The contestant is given 3 doors to choose from. Behind one door is a new car and behind the other two are goats. The contestant chooses one door. The host then shows the contestant a goat he knows to be behind one of the other doors. The contestant is then given the opportunity to switch his choice. What should the contestant do? Well, given that he is not a shepherd and wants the car the odds say that he has a 66% chance to win the car if he switches his choice. This doesn't seem to make sense as there are only two doors left, so the odds should be 50-50. However, as the problem was originally explained by Marylin vos Savant (the woman with supposedly the world's highest IQ) in a Parade magazine letter response which drew some 10,000 digressing responses from readers, some of whom were mathematicians and professors.
The explanation follows:

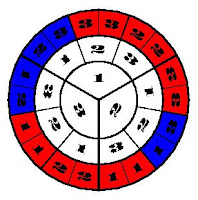
To analyze this problem we represent this senario as a random variable on a roulette wheel. The roulette wheel on the left simulates the Let's Make a Deal game. The inner wheel represents the number of the door that the car is behind, the middle wheel represents the door that is selected by the contestant, and the outer wheel represents the door Monty Hall can show. Spinning this roulette wheel once is equivalent to playing the game once. The outer wheel also tells you what your strategy should be to win. The red means that in order to win the contestant needs to switch doors, and the blue means that the contestant should not switch. Notice that there are twice as many red sections as blue. In other words, you are twice as likely to win if you switch than if you don't switch! What this wheel makes evident is that with probability 1/3 the contestant selects the correct door in which case it would be better not to switch. In the other 2/3 of the cases, Monty Hall is telling the contestant where the car is!

I still can't seem to wrap my head around this explanation, but the evidence confirms it. If you manually go through the game enough times and record the outcome of the choices you will find switching yields about a 66% success rate. Too bad the show is off the air.